In the video below, I explain how to derive Kaluza Klein action which occurs from generalizing the Einstein-Hilbert action to five dimensions. The grand implication of this is that gravity and electromagnetism are unified!
Tag: science
Abstract: It is shown that the standard/common definition of team offensive rating/offensive efficiency implies that a team’s offensive rating increases as its opponent’s offensive rebounds increase, which, in principle, should not be the case. Over the past number of years, the advanced metric known as Offensive Rating has become the standard way of measuring a basketball […]
Here is an embedded dashboard that shows a number of statistical insights for NBA teams, their opponents, and individual players as well. You can compare multiple teams and players. Navigate through the different pages by clicking through the scrolling arrow below. (The data is based on the most recent season “per-game” numbers.) (If you cannot […]
As more and more teams are increasing the number of threes they attempt based on some misplaced logical fallacy that this somehow leads to an efficient offense, we show below that it is in fact in a team’s opponent’s interest for a team to attempt as many three point shots as possible. Looking at this […]
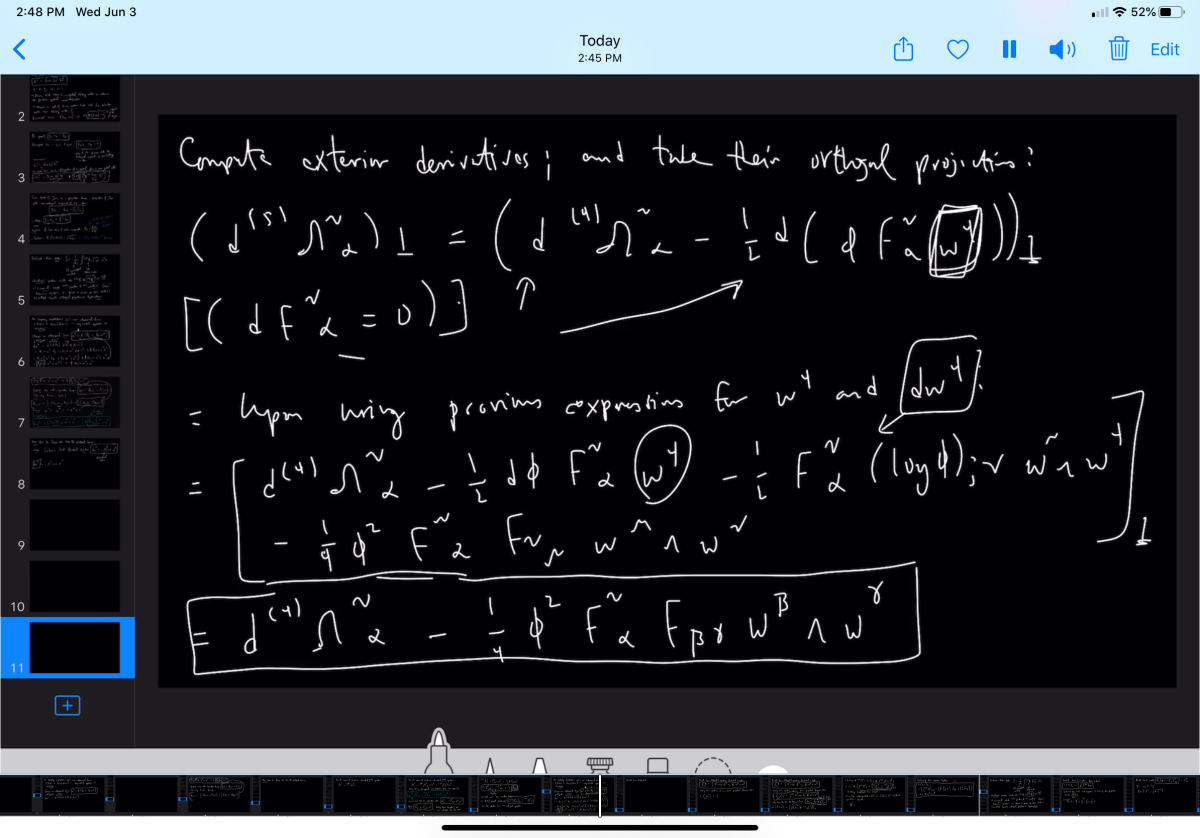
Our new article was recently published in The Journal of Geometry and Physics. It is shown that under certain conditions, The Einstein Field Equations have the same form as a fold bifurcation seen in Dynamical Systems theory, showing even a deeper connection between General Relativity and Dynamical Systems theory! (You can click the image below […]
Here is a link to my lectures on nonlinear dynamical systems given at York University during the Winter semester of 2017. These lectures start off with manifold theory, and end with examples in biology, game theory, and general relativity/cosmology.
Our new paper examining the dynamics of an asymmetric Hawk-Dove game was published in The International Journal of Differential Equations:
In the final two lectures of my differential equations class , I discussed how Dynamical Systems theory can be used to understand and describe the dynamics of cosmological solutions to Einstein’s field equations. Videos and lecture notes posted below: Lecture Notes:
I have now made a significant update to my applied machine learning paper on predicting patterns among NBA playoff and championship teams, which can be accessed here: arXiv Link .
New #cosmology paper: https://arxiv.org/pdf/1609.01310.pdf Using a dynamical systems approach to provide a unifying framework for the AdS, Minkowski, and de Sitter universes. #physics #mathematics #science
Relativity Digest 